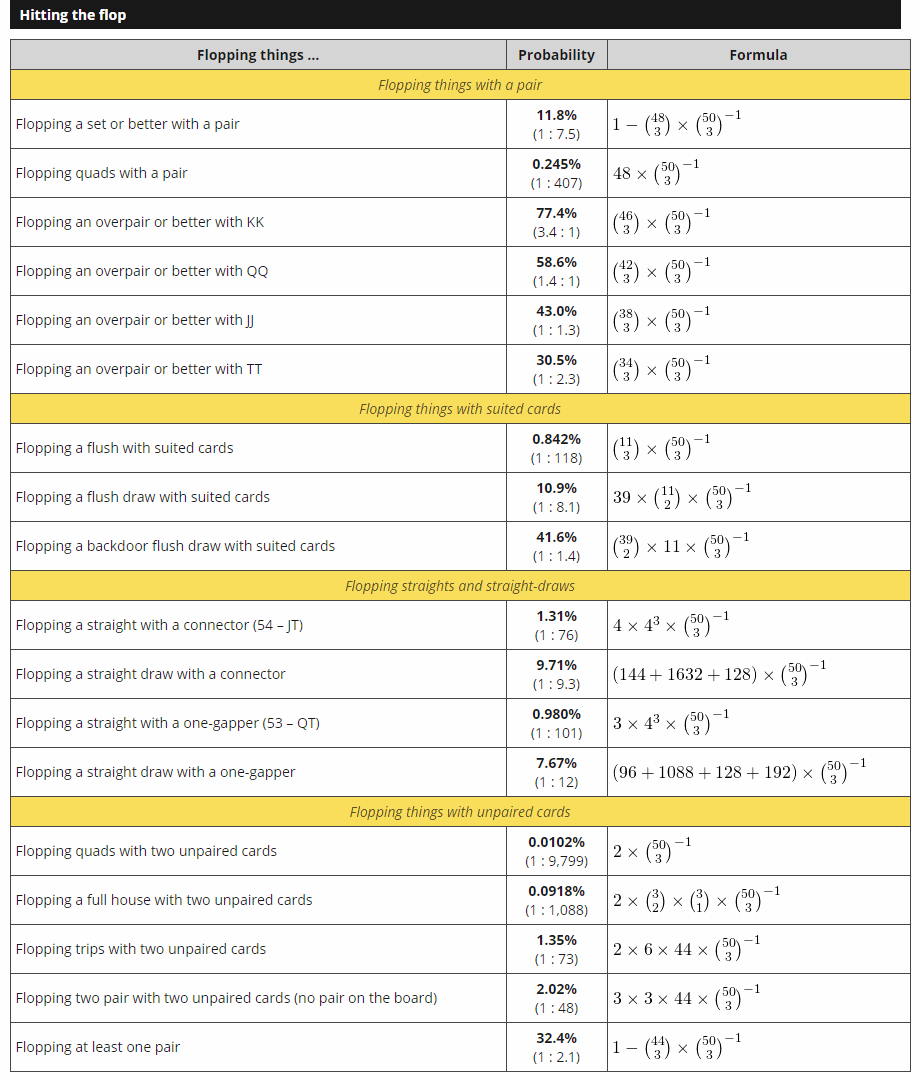
Odds Of Flopping A Set
- In fact, the math suggests that somebody will flop a higher set than you in a full ring cash game once every 7.8k hands. It is even more rare in a 6max game, where it will only happen once every 12.6k hands. This is why set over set is commonly referred to as a 'cooler'.
- Odds of flopping 2 Pair or better with an unpaired starting hand = 4.1%. So, although it’s relatively unlikely we’ll flop exactly 2 Pair, we’ll actually flop Two Pair or better roughly once in every 25 flops we see. Unlike flopping Two Pair, the Two Pair plus odds, do change based on the type of hand.
The question is - what are the odds of flopping a set? When you hold a pocket pair, the odds of flopping a set are 7.5-1, or roughly 12%. It's important to know the odds, as many people will try to 'set mine' when playing Texas Hold'em. This means that you are calling pre-flop bets with your pocket pair, hoping to hit a. Odds Percent; Double wraparound straight draw (e.g. Hand: 9-8-5-4, flop: 7-6-x) 0.48-1: 68%: Wraparound straight draw (e.g. Hand: 8-5-4-x, flop: 7-6-x) 0.67-1: 60%: Straight flush draw: 0.84-1: 54%: Hitting a full house with three pairs: 3-1: 24%: Hitting a full house with two pairs: 5.1-1: 16.5%: Hitting quads with a set. The odds of flopping a set when holding a pocket pair is approximately 12%. The odds of flopping a set when holding a pocket pair AND somebody else flopping a set while holding a pocket pair is roughly 1% (the actual number is a bit over 1%). So if you feel are though you took a really bad beat after losing in a set-over-set situation, you most certainly did, as you had a 1% chance of losing in that fashion when.
My question is: what are the odds of flopping a set with the same pocket pair 3 times in a row?
The wizard answered a previous question about what are the odds of getting the same pocket pair 3 times in a row(.000142108 or 1 in 7037):
/forum/gambling/poker/31884-odds-of-same-pocket-pair-three-times-in-a-row/
So would I take this number and multiply it by the chances of flopping a set 3 times(.12 * .12 * .12 = .001728)? When I multiply those numbers together I get .00000024192 or 1 in 4,133,598.
By the way, I did come back from an 8 to 1 deficit to win the game, mostly due to those 7s!
The craziest thing happened to me while playing a home game online last night. I was heads up with a buddy of mine and was severely short stacked(I had around 8K he had around 65K). I got dealt pocket 7s and went all in and he quickly called with Queens. I hit a set on the flop to stay alive. Next hand I get 7s again and go all in and he calls with Q8. He hits the queen on the flop but I hit a set of 7s on the flop again to win the hand. The very next hand I get pocket 7s again and raise, my buddy calls. I flop a set of 7s a third time! When we get to the river, I win against his AK(he hit a K on the flop to stay in the hand).
My question is: what are the odds of flopping a set with the same pocket pair 3 times in a row?
The probability of getting a third card matching a pocket pair in the flop is 1 - (48/50 x 47/49 x 46/48) = 144 / 1225.
The probability of getting the same pocket pair in 3 consecutive hands is 1/17 (the probability of getting a pocket pair in the first hand) x 1/221 (the probability of getting the same pocket pair in the next hand) x 1/221 (the probability of getting the same pocket pair in the third hand) = 1 / 830,297.
OOPS - corrections are in red
The overall probability = (144 / 1225)^3 x 1 / 830,297 = about 1 / 511,000,000
Note that this applies to three specific hands, and not necessarily any three consecutive hands in a session.
Odds Of Flopping A Set In Plo
Assuming you are dealt a pocket pair, the probability of getting a third card on the flop and then being dealt the same pair and getting a third card on the flop in both of the next two hands is this multiplied by 17, or about 1 / 30,000,000
1 / 30,000,000The probability of getting a third card matching a pocket pair in the flop is 1 - (48/50 x 47/49 x 46/48) = 144 / 1225.
The probability of getting the same pocket pair in 3 consecutive hands is 1/17 (the probability of getting a pocket pair in the first hand) x 1/221 (the probability of getting the same pocket pair in the next hand) x 1/221 (the probability of getting the same pocket pair in the third hand) = 1 / 830,297.
The overall probability = 144 / 1225 x 1 / 830,297 = about 1 / 7,063,290.
Note that this applies to three specific hands, and not necessarily any three consecutive hands in a session.
Assuming you are dealt a pocket pair, the probability of getting a third card on the flop and then being dealt the same pair and getting a third card on the flop in both of the next two hands is this multiplied by 17, or about 1 / 415,488.
you need to cube the 144/1225 term, no?
Why, yes - yes, I do. Corrections to the original post have been made.
Odds Of Flopping A Flush
Thanks for the detailed response! So for the purposes of bragging, I can say that this was a 1 / 511,000,000 chance of happening then? In other words, I had a better chance of winning Powerball and will probably never see it again?
1 / 511,000,000 is for three specific hands. If you're talking three consecutive hands in general when you have been dealt a pair, it's 1/17 of that, or about 1 / 30,000,000.
Odds Of Flopping A Set When Dealt A Pair
Chances of hitting, flopping and holding certain hands
These odds are a must know if you want to advance your game to a high level. For exact odds you can check out our poker hand odds calculator. We rounded the number to the nearest decimal for you.
+2+cards+(Turn+%26+River).jpg)
You should know what beats what in poker before trying to apply these odds or playing like you see poker on tv and in commercials.
| Scenarios – Chances of Having Certain Hands | Examples | Probability | ||
| Chances of Being Dealt | ||||
| Pair | 6h 6d | 6% | ||
| Suited Cards | Ah 10h | 24% | ||
| Connecting Suits | 2d 3d | 4% | ||
| Aces or Kings | AA KK | .9% | ||
| Ace King | AhKs | 1.2% | ||
| Ace King Suited | AhKh | .3% | ||
| An Ace | A3 | 16% | ||
| Cards Jacks or Higher not Paired | KJ | 9% | ||
| Not Suited & Not Connected | 9h 4s | .9% | ||
| Bad Beats | ||||
| Bad Beat ex: Aces vs Kings heads up | AA vs KK | .004% | ||
| Chances of Hitting on Flop | ||||
| Pocket Pair Into A Set | JJ into JJJ | 8% | ||
| Pair Turning Into A Set On Turn | 4% | |||
| Hitting Pair on Flop | 32% | |||
| Flopping Four To Flush-You hold 6h7h-flop comes-> | Ah Kh 2s | 11% | ||
| Chances of Board Coming All Same | 5h 5s 5d | .004% | ||
| Number of Players To Flop Odds | ||||
| Situation – Chances someone hit top pair on board | ||||
| 5 players see flop | 58% | |||
| 4 players see flop | 47% | |||
| 3 player see flop | 35% | |||
| 2 player see flop | 23% | |||
| After Flop – Chances of Making Hand | ||||
| Making open straight – You hold 67 Flop comes 8,9,2 | turn 10 | 34% | ||
| Two pair to full house – You- 47 Board 4,7,10 Turn –> | 7 | 17% | ||
| Hitting A Gut Shot Straight | 17% | |||
| Backdoor Flush – You have 1 spade – Board 2s4h8s | 10s 7s | 4% | ||
| Runner Runner Straight | 1.5% | |||
| Hitting Either Gut Shot Straight or Backdoor Flush | 21% | |||
| Pairing An Ace on Turn or River | 13% | |||
| Before Any Cards Are Dealt – Chances of Getting | ||||
| Royal Flush (All Spades) | AKQJ10 | .0002% | ||
| Straight Flush (Any same suits) | 56789 | .0012% | ||
| Four of a Kind (Quads) | 5555K | .0239% | ||
| Full House (Boat) | 33322 | .144% | ||
| Flush (all same suit) =>all hearts | 37K48 | .19% | ||
| Straight | 34567 | .35% | ||
| Three of a Kind | 555AK | 2.11% | ||
| Two Pair | AAKK2 | 4.7% | ||
| One Pair | 77253 | 42% | ||
| Don’t catch anything | 2854K | 50% | ||
Why Poker Odds Matter
Why Odds Matter To any good Texas Holdem players these odds come naturally. They may not know the exact percentage but they instinctively know their odds. Referencing this table is a great way to understand your percentages if you are a new player or if you want to calculate your pot odds.
We developed what we believe are the best formulas for calculating pot odds that you will find on the internet. It is the same way the pros calculate their pot odds and we also simplified it for those of you who are not that good at math. Check out the Pot Odds section.